Second-Order Embedded Julia Set
Robert P. Munafo, 2023 Jul 7.
Truly the most stunning features of the Mandelbrot Set, when viewed with the aid of the Distance-Estimator function, are the higher-order Embedded Julia Sets.
Here is an example (click on it to enlarge):
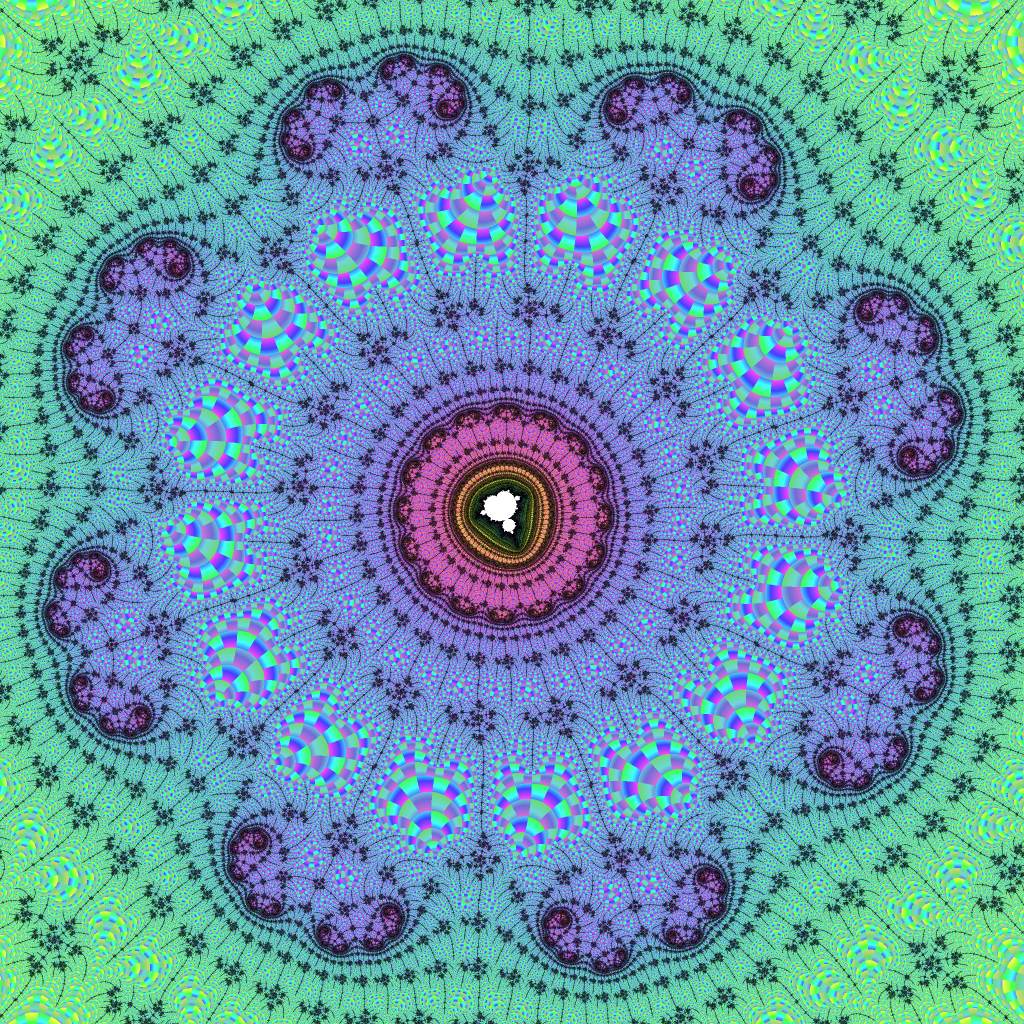
A second-order embedded Julia set
Just as Embedded Julia Sets are found by exploring very close to an island Mu-molecule, the second-order Embedded Julia Sets are found by exploring very close to an island — namely, the island at the center of a first-order embedded Julia set, or an island at the center of a paramecium.
In the term "second-order embedded Julia set", the order indicates how many islands you have gone close to while zooming in. An island counts only if it is not part of the mu-unit of a larger island which has already been counted. Every new mu-unit you get close to introduces a new "distortion field" (more accurately, tuning) that creates Julia Sets, and the place you explore in relation to this mu-unit determines the shape of the Julia set for that order. Each time you go to a higher order, another Julia Set shape is added to the nested symmetric filaments around the island at the center.
Dense and Sparse
Once getting in to the center of the first embedded Julia set, one can select a new target very close to the central island to find a second-order embedded Julia set like that pictured above and exemplified in the zoom sequence below (where the new target is found within the Elephant Valley of the central island). However one could also select a mitochondrion, node or paramecium (a new target fairly far from the central island) which is effectively a very sparse Fatou dust, and arrive at a new central island surrounded by repeated shapes of whatever mito/node/paramecium was chosen.
Higher Orders
There are of course third-order, fourth-order etc. embedded Julia sets. Ordinarily I try to restrict this term to those that have a fairly dense Julia set for each new higher order; however there is no clear boundary between dense and sparse. The popular art of Leavitt navigation for deep zooms usually picks new targets several or many times, allowing for a very diverse range of designs combining many fractal forms.
Zoom Sequence
During the course of this sequence we encounter tuning and non-tuning Embedded Julia Sets. The atom domain views (the right image in each pair) show which of the embedded Julia sets represent a new tuning period. Tuning changes twice: when the color changes from green to yellow; and then later when it changes from yellow to blue.
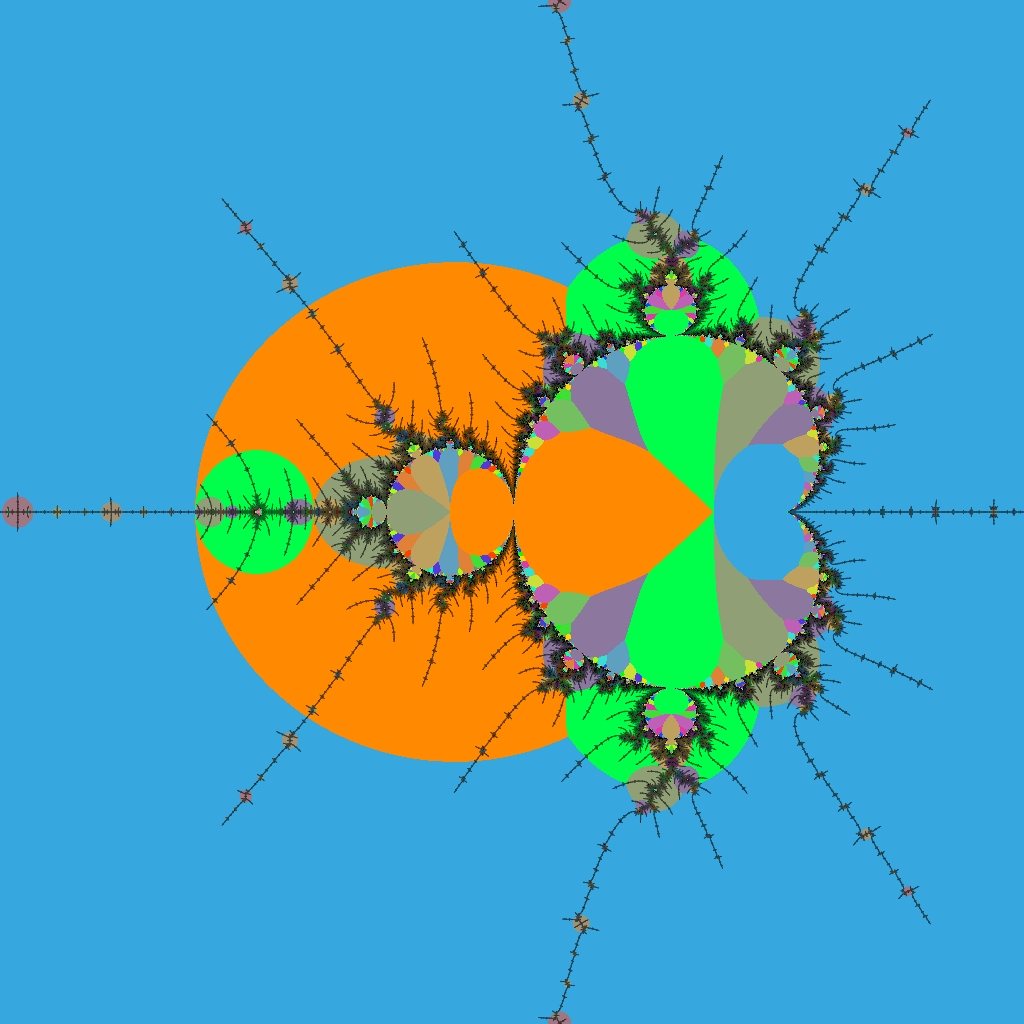
 | 
|
This is the period-3 island R2F(1/2B1)S. On the left is an ordinary view combining dwell, binary decomposition and distance estimator information showing the filaments.
On the right we see atom domains. Period-3 (the period of R2F(1/2B1)S) is blue; period 6 is orange; period 9 is green, and so on.
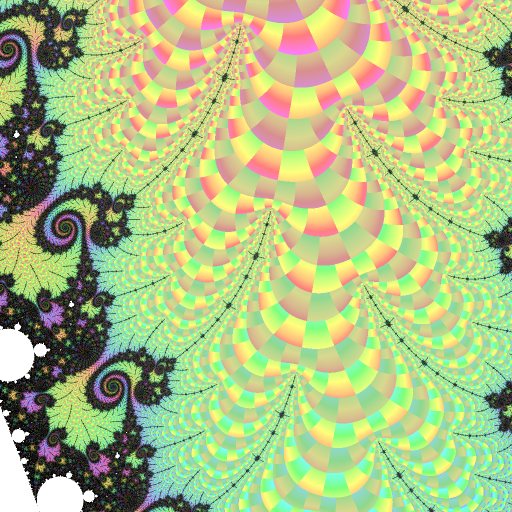 | 
|
Here we see some filaments within the "Seahorse valley" area of R2F(1/2B1)S. The R2-name of this cusp is R2F(1/2B1)S.C(1/2)-.
In the atom-domains image we see some higher periods near the left side, but most of the image is dominated by the orange of the period-6 mu-atom R2F(1/2B1)S.1/2a.
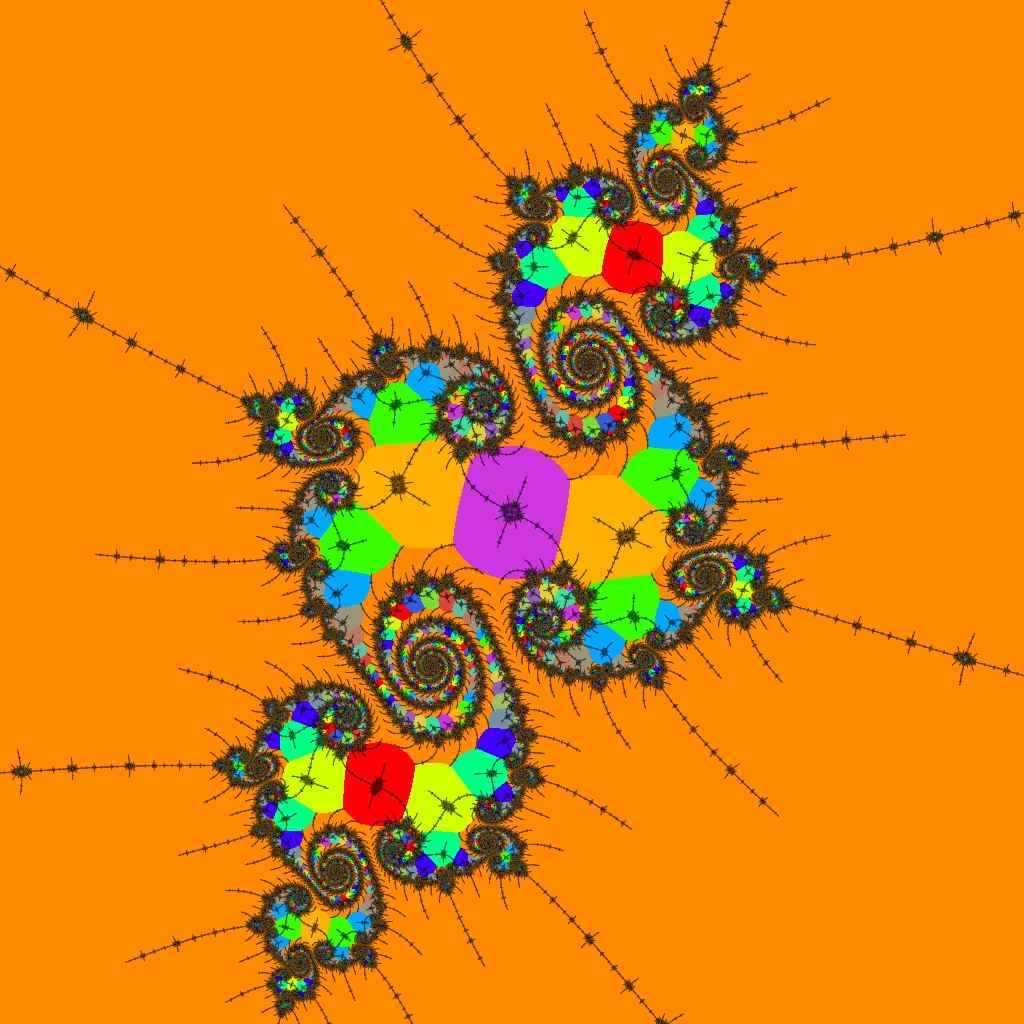
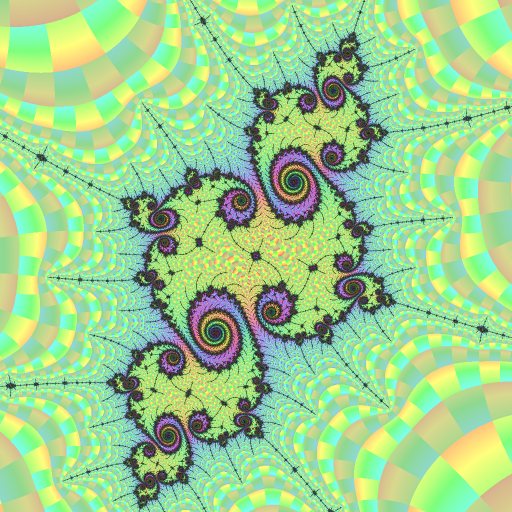 | 
|
This is a first-order, 2-fold embedded Julia set within R2F(1/2B1)S.C(1/2)-.
In the normal DEM-dwell hybrid view on the left, contrasting color highlights show where the dwell values increase significantly at the boundary of the embedded Julia set.
Many new colors are seen in the atom-domain image on the right, showing the locations and periods of the embedded Julia set's nucleus and paramecia. As we continue to zoom in, this image will be mainly purple, the color corresponding to the period-101 mu-molecule at the center of the 2-fold embedded Julia set.
 | 
|
This is the 4-fold nucleus of the embedded Julia set. The dwell values change significantly (seen by the distinct difference in color between the inside and outside of the nucleus), but the right image is dominated by the solid purple of a period 101 atom-domain.
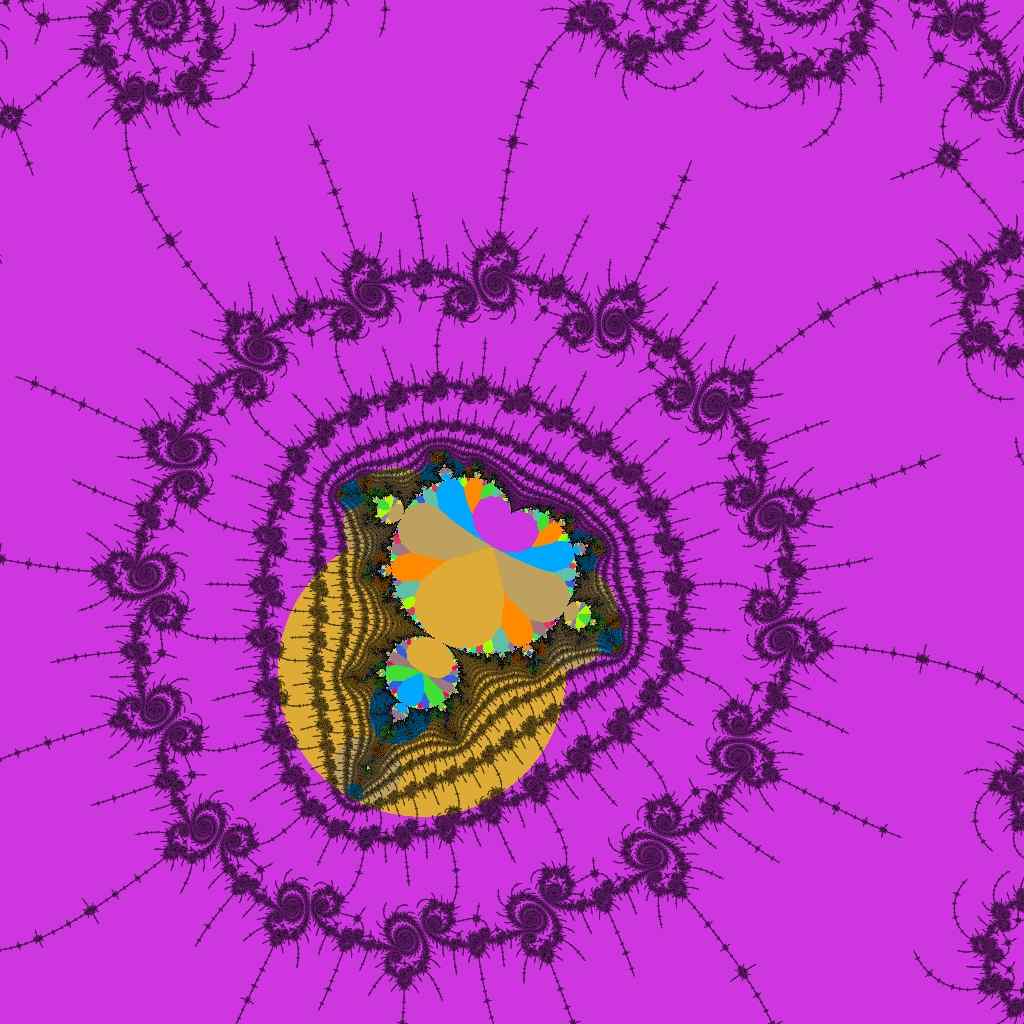
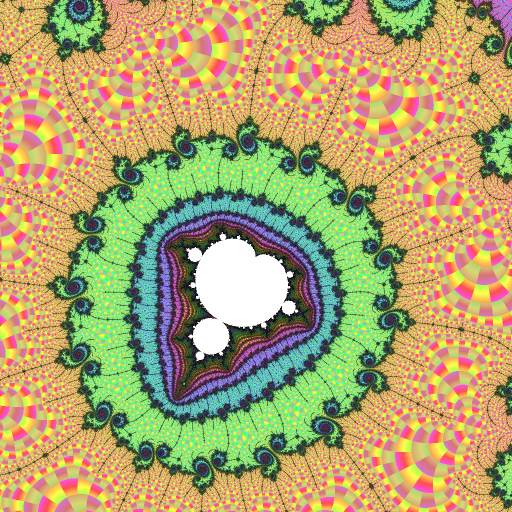 | 
|
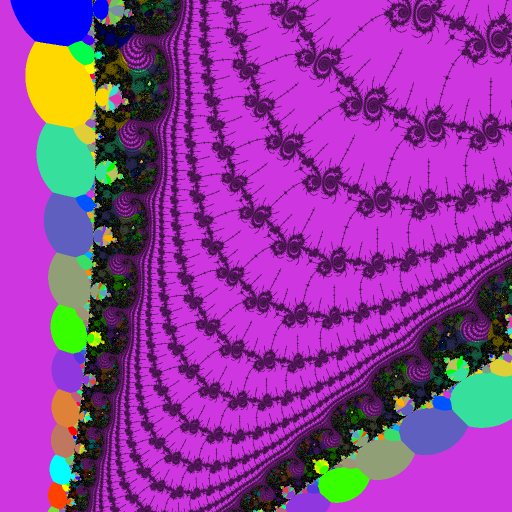
Now we see the period-101 island. As with R2F(1/2B1)S, this island "tunes" the area around it, creating a nested concentric series of rings of features, doubling in number at each stage.
There are also new atom-domain colors in the right-hand image; each domain has a mu-atom whose period is a multiple of 101: salmon for period 202, brown for period 303, etc.
 | 
|
Here we approach the elephant valley or .C(0) cusp region of the period-101 island.
 | 
|
In these views there is plenty going on in the filaments, but little color contrast in the dwell values on the left. On the right, everything is purple because the strongest tuning is still that of the period-101 island (which is completely surrounding us, though it is now out of sight).
 | 
|
This 2-fold embedded Julia set, is an "echo" of the original 2-fold embedded Julia set (seen above at size 4.5×10-6). The dwell values do not change significantly (resulting in lack of contrasting colors in the left image), and there are no new atom-domains in the right image.
 | 
|
A 4-fold "echo" of the original embedded Julia set. Again there is no significant contrast and the dominant period is 101.
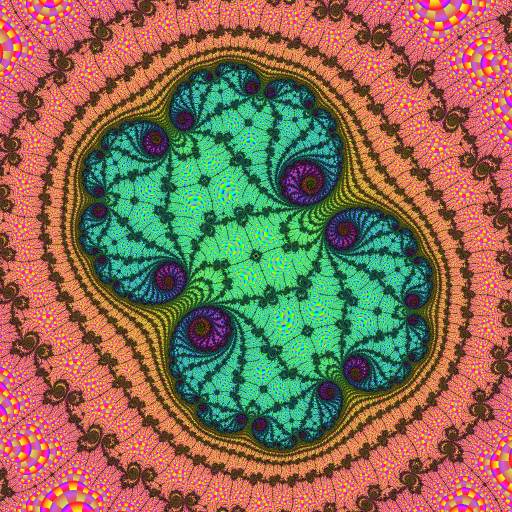 | 
|
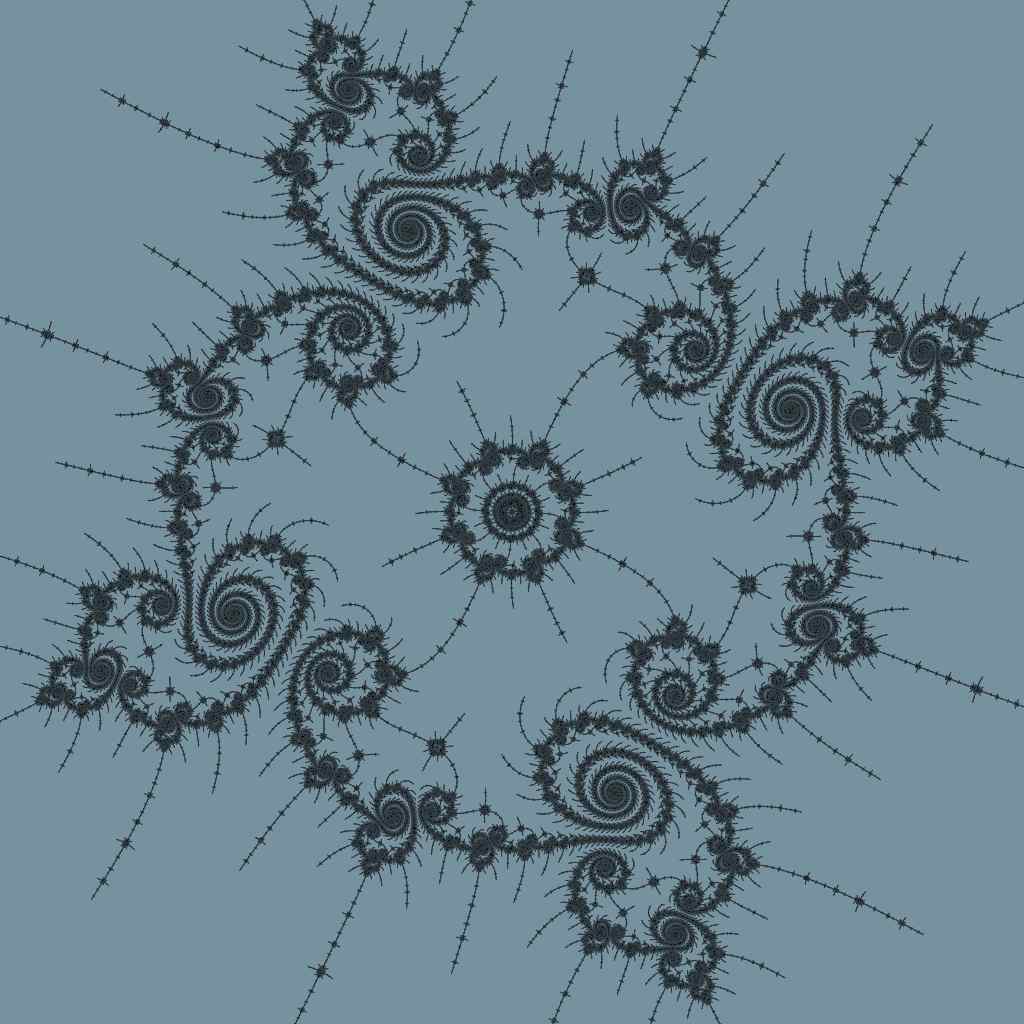
Now we arrive at the second-order embedded Julia set. Significant color contrast makes it stand out in the dwell view on the left.
Unlike the "echo" embedded Julia sets above, this time there are lots of new atom-domains. These show the locations and periods of the nucleus and paramecia. As we proceed further the dominant period will be 2123, the period of the influencing island of this second-order embedded Julia set. This period shows up as gray in the right-hand image.
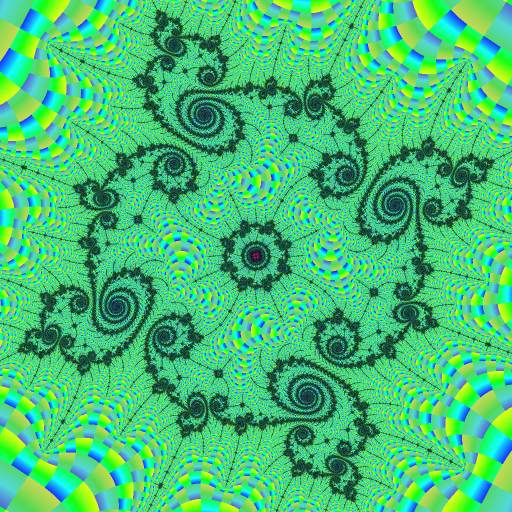 | 
|
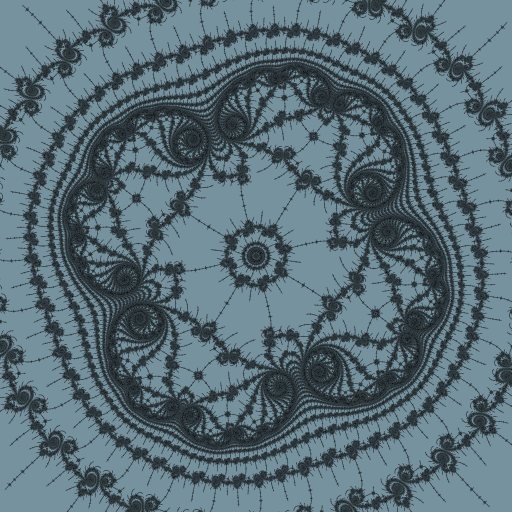
Another 4-fold "echo" of the original embedded Julia set. Here there is no contrast in either view. The atom-domain image is the dull gray of period 2123.
 | 
|
This is the 4-fold symmetric nucleus of the second-order embedded Julia set. This time the dwell values jump significantly (seen by the contrasting colors in the left image) but there is no strong local tuning (evident from the image on the right having just one color).
 | 
|
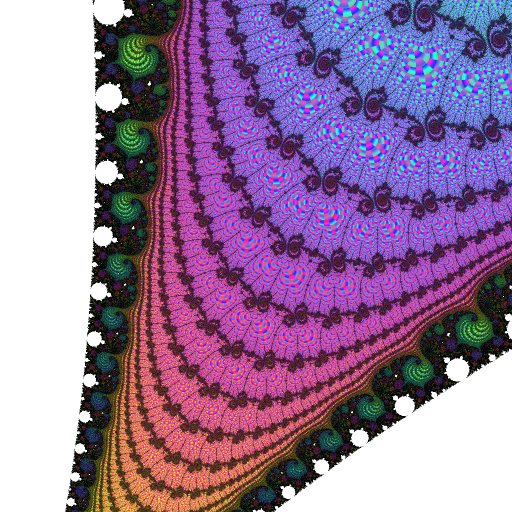
Now we arrive at the period-2123 island at the center of the second-order embedded Julia set.
Here each of the concentric binary rings that approach the island as their limit has its own set of binary rings.
In the right image, atom domains of its child mu-atoms can be seen: the period-4246 .1/2a (red), period-6369 .1/3a (purple), etc.
See also second-order satellite, 2-fold embedded Julia set, n-fold embedded Julia set.
revisions: 20020418 oldest on record; 20120421 add zoom sequence; 20230707 dense/sparse; higher orders; images have new colors
From the Mandelbrot Set Glossary and Encyclopedia, by Robert Munafo, (c) 1987-2024.
Mu-ency main page — index — recent changes — DEMZ
This page was written in the "embarrassingly readable" markup language RHTF, and was last updated on 2023 Jul 08.
 s.27
s.27